Robert John Aumann (Hebrew name: ישראל אומן, Yisrael Aumann; born June 8, 1930) is an Israeli-American mathematician and a member of the United States National Academy of Sciences. He is a professor at the Center for the Study of Rationality in the Hebrew University of Jerusalem in Israel. He also holds a visiting position at Stony Brook University and is one of the founding members of the Stony Brook Center for Game Theory. His website is here.
Prof. Aumann received Nobel Prize in Economics in 2005 for his work on conflict and cooperation through game-theory analysis. He shared the prize with Thomas Schelling. You can find his complete Nobel lecture on the incentives which lead nations to War and Peace here.
In the following series of video lectures, Aumann makes some very interesting connections between the Talmud and modern economic theory. Prof. Aumann’s original slides from the lecture are available here.
Fixing the World: Exorbitant Ransom
The first topic is related to Prof. Aumann’s prize-winning research in the area of incentives in game-theory. Game-theory is a study of strategic decision making. Specifically, it is “the study of mathematical models of conflict and cooperation between intelligent rational decision-makers”.
Rational decision makers in game-theory prefer outcomes that maximize their gain (or minimize their loss). A person’s behavior is considered rational if his actions are in his best interests, given his information. This doesn’t mean that there is no place for altruistic motives, but we would still like to avoid negative incentives which discourage people from “doing the right thing”.
At 7:10 minutes in the video, prof. Aumann explains that in the Talmud “Tikkun Olam” (תיקון עולם), which literally means “Fixing the world”, always refers to a law (Halacha) which needs to be amended because it empirically creates negative incentives. Even though the law was intended to have a positive effect on society, the negative incentives it creates result in a negative outcome for society: people find it difficult to “do the right thing”, or are incentivized to “do the wrong thing”.
Aumann mentions Gittin Chapter 4, which has several examples of this principle. One example deals with paying exorbitant ransoms for captives:
“No more money must be paid for the redemption of captives than what they are really worth, due to tikkun olam.”
As Aumann explains, paying exorbitant ransoms creates a negative incentive. Although redemption of captives (פדיון שבויים) was meant to be a humanitarian law, in reality it was found that paying exorbitant ransoms creates incentives for criminals to kidnap. We see this in modern times when Israel’s willingness to free 1,000 prisoners for Gilad Shalit has created an incentive for Hamas to try and kidnap more Israeli soldiers. This is what Aumann means by negative incentives, and removing such incentives is what the Talmud means by “Tikkun Olam”.
Aumann even brings a reference from Maimonides which explicitly states that “Prisoners should not be redeemed for unreasonably high ransoms, so that enemies should not pursue people to kidnap them.” (Maimonides, Codex, Laws of Charity, Chapter 8, Section 12).
Fixing the World: Prozbul
In the next segment, Prof. Aumann continues an example from Part 1 about modern day Vietnam, and then explains topic of Prozbul.
The Torah mandates a Sabbatical year, known as Shmita, every seventh year, which, among other things, cancels all debts. This is one of the many laws in the Torah meant to protect the poor and disadvantaged, affording them a chance to escape from eternal debt. But, Aumann explains, when applied in practice, the law created an unforeseen negative incentive: lenders stopped lending as the 7th year approached, because they did not want to lose their money. This had a negative effect on the economy and on the poor, and so Hillel found a way to effectively cancel the law by assigning the debt to someone else for a year and reinstating it thereafter.
Price Control and Competition
At 3:45 minutes, Aumann starts his second example: price control and competition. He starts by quoting the biblical source (Deutoronomy 25):
The Talmudic discussion on this topic takes place in Bava Batra 89:
The sage Shmuel tells his assistant named Karna (literally: Horn) to appoint supervisors to oversee the fairness of scales and measures in the marketplace but not to oversee the fairness of prices. Karna disobeys him and proceeds to enforce “fair” prices, and Shmuel curses him that he may grow a horn between his eyes (hence the playful name “unicorn” for this topic in Aumann’s slides).
Aumann claims that the Talmud was in favor of government regulation of scales and measures, but against centralized price controls. In other words: create incentives to keep the merchants honest, but don’t create incentives for fixing prices, because the free market already has the right incentives built-in: competition. The argument continues in part 3:
Aumann gives the commentary of the Rashbam (Rashi’s grandson, who replaced his commentary when he died in the middle of Bava Batra) which states that if one person sells for an exorbitantly high price, then there is an incentive for someone else to sell at a lower price, and the buyers have an incentive to buy from that person, which will force the first seller to lower his prices as well. Here is a hebrew script of the commentary:
Aumann’s translation is as follows:
At 4:00 minutes, Aumann makes the point that while you may argue that the economics of free market prices are only implicitly favored in the Talmud, you cannot deny that the idea that the market will “self-regulate” its prices is stated very explicitly in the Rashbam commentary (which dates back to the 12th century).
This concept of the “invisible hand” that guides the market to fair pricing is usually credited to Adam Smith in his 18th century classic The Wealth Of Nations.
Moral Hazard (Ten Stores)
At 7:20 minutes, Aumann starts his next example: moral hazard. He brings a famous story which is repeated multiple times in the Talmud. This excerpt is from Ketubot 15:
In English: “There are 10 stores, all selling kosher meat, except one, which is selling non-kosher meat. If a man buys from one, but doesn’t remember which one, then due to the doubt, the meat is forbidden; but if he found the meat, we go by the majority.”
In the 4th segment, prof. Aumann explains this case using the modern concept of moral hazard. A risky situation is said to be fraught with “moral hazard” if the outcome is determined (or may be affected) by the actions of an interested party.
The example Aumann gives to illustrate “moral hazard” is this: a person wants to buy insurance for his house which is worth $500K. He pays the premium, and then offers the insurance company to buy two policies, each for $500K. Any rational insurance company would have to refuse. Why? Because it’s a “moral hazard”: the person would then have an incentive to burn down his house, because the payoff from insurance is higher than the payoff from selling. This gets back to Aumann’s idea that real-life decisions are influenced by positive and negative incentives: “moral hazard” can occur whenever your own actions influence the probability that an adverse event will occur. This is a problem for insurance companies, because their premiums are calculated based on the assumption that there is incentive for the owner to keep his house safe.
Getting back to the Talmud, Aumann explains why this is a classic “moral hazard” situation: the person who bought the meat forgot at which store he bought it. But since he was the one who bought it, and he already spent the money, then, consciously or subconsciously, he has an incentive to think that he probably bought it at the kosher store, because then he won’t have to throw it away. This is the essence of the moral hazard: when results of your own actions influence what is supposed to be an independent probability calculation. Some may be able to resist the incentive to cheat, but others may not, so the Talmud takes that into account and removes the incentive.
This is why the Talmud adds the second case: suppose he found the meat. In that case, the choice of which store the meat came from was not in the person’s own hands, so the probability can be computed objectively, and in this case there is a 9/10 (90%) chance that the meat is kosher, so the person can eat it (“go by the majority” means more than 50% probability).
Median Voting (Multiple Appraisers)
One example that is in prof. Aumann’s slides, but not in this particular video (there are many other lectures of his online) is the case of multiple appraisers: if you get 3 appraisals for your house, should you always take the average or the median?
The surprising game-theory answer is given by the Median Voting Theorem: “a majority rule voting system will select the outcome most preferred by the median voter”. The median rule turns out to be the optimal aggregation strategy for single-peaked preferences, as explained by the following video:
The wikipedia article about the median voter theorem gives a simple example to illustrate the idea, but prof. Aumann found even simpler examples in the Talmud.
Aumann’s English translation and explanation is as follows:
The modern-day explanation is simply that in both cases we select the median, rather than the mean, but the Talmud and commentary bring logical arguments. The latter case is actually the simplest. If one says 100, one says 80 and one says 120, then we pick the average which is 100. But what if two of them agree? In that case, the average leaves the majority unhappy. So, the Talmud adds the first part: if any two of the appraisers agree, then they choose by the majority. This is the same result as would be given by the median voter theorem.
The Three Widows (Consistent Fair Division)
This example is one of prof. Aumann’s most well-known and complicated results. He was the first modern scholar to show that the Talmud’s solution can be explained with modern game-theory.
The problem and the solution are stated in Ketubot 93a (and let’s dodge the issue of how a man can have 3 wives, and why he promises them different inheritances in their ketubot):
“If a man with three wives dies, one has a ketuba of 100 zuz, one of 200, and one of 300, and there is only 100 in the estate, then they divide equally.
If there is 200, then the one of 100 takes 50, and those of 200 and 300, 75 each.
If there is 300, then the one of 100 takes 50, the one of 200 takes 100, and the one of 300 takes 150.”
In the following diagram, the amounts promised to each wife are shown on the top row and the amounts available for inheritance are shown the left column. Each inner cell then shows how much each widow would get in the 3 cases where the deceased left 100, 200 or 300 respectively:
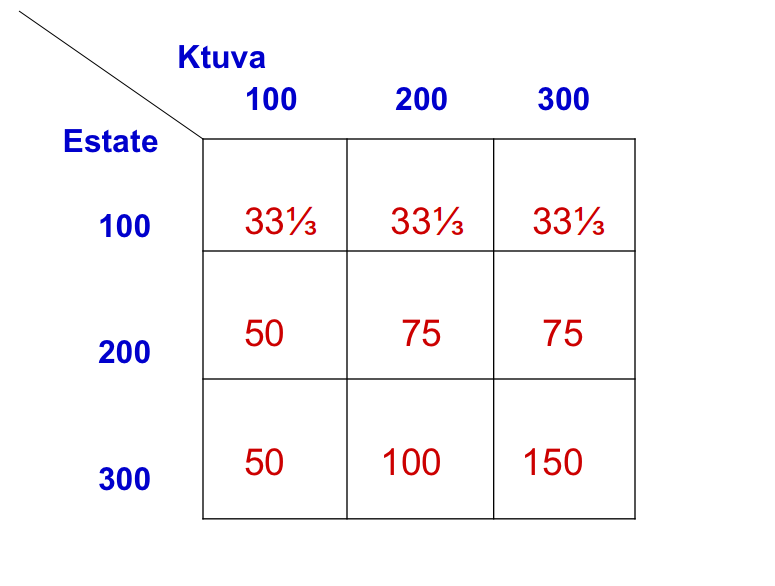
The last case, where the man left over a sum of 300, is to be divided according to the ratios of the promised amounts. This seems obvious. But why is the first case divided equally? And why is the second case divided 50 to the first and then 75 each to the other two? At first glance, this seems inconsistent. Indeed, this has been a mystery for centuries until prof. Aumann provided the mathematical explanation.
You can read prof. Aumann’s paper, which explains why the solution in the Talmud is consistent with game-theory, and watch him explain it in the next video segment: